Groundbreaking Discovery in Mathematics

In a stunning revelation that has sent shockwaves through the world of mathematics, researchers have debunked the once widely accepted "Bunkbed Conjecture." Initially thought to be a fundamental truth within probability theory, this conjecture has been proven wrong, sparking discussions about the nature of mathematical conjectures in general.

Understanding the Bunkbed Conjecture

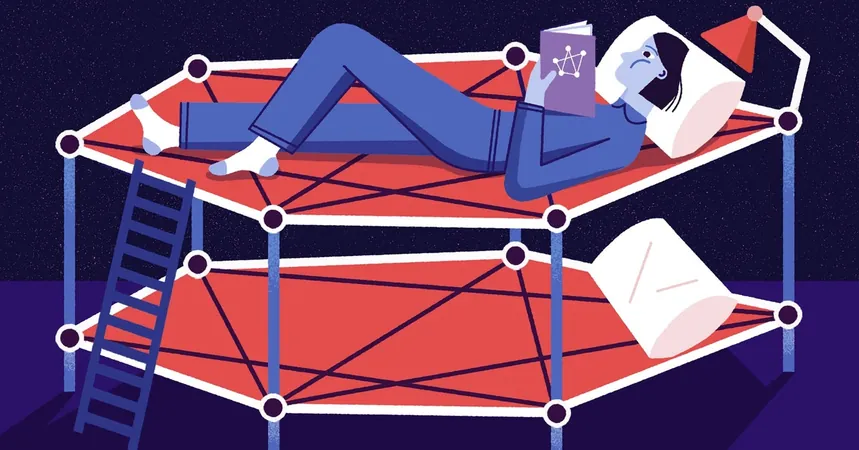
The conjecture itself was based on a graphical structure representing two identical graphs laid one above the other, resembling a bunk bed. Vertically connecting these two structures involved drawing additional lines, or "edges," between corresponding vertices. The conjecture suggested that, after performing random eliminations of these edges based on a simple coin flip, certain properties would persist regardless of the random processes applied. Yet, this bold assertion was held suspect by some prominent mathematicians.

Skepticism Becomes Reality

Igor Pak, a mathematician from UCLA, harbored doubts about the Bunkbed Conjecture from the very beginning. Known for challenging established ideas, Pak, along with his graduate student Nikita Gladkov, embarked on a quest to uncover the truth. While many in the field rushed to prove conjectures as valid, Pak questioned whether it was time for mathematicians to consider the possibility that long-held beliefs might be fundamentally flawed.

Over the course of a year, Pak made significant attempts to disprove the conjecture but faced failures. Realizing the complexity of the task required more computational power, they turned to their friend Aleksandr Zimin from MIT, who brought much-needed expertise to their research. They employed computational methods and neural networks to provide statistical support for their challenge of the conjecture, but doubts lingered about their findings' acceptance among skeptics of computational proofs.

An Unexpected Turn of Events

The turning point came unexpectedly in June when Lawrence Hollom at the University of Cambridge tackled a similar problem, adjusting the focus from standard graphs to hypergraphs. In hypergraphs, edges don’t just connect pairs of vertices—they can link multiple vertices simultaneously. This broader context allowed Hollom to uncover a counterexample that refuted the conjecture.

He meticulously crafted a small hypergraph where each edge connected three vertices. This counterexample not only challenged the foundations of the Bunkbed Conjecture but also opened new avenues of understanding regarding hypergraphs and their complex interconnections.

The Significance of the Discovery

This groundbreaking work highlights the importance of adaptability in mathematical theory and the necessity of challenging established ideas. While the Bunkbed Conjecture will be remembered as a fascinating chapter in the study of probability and graph theory, its debunking paves the way for future explorations that could redefine mathematical boundaries.

As mathematicians around the globe re-evaluate their approach to conjectures, this development prompts a crucial question: How many other long-held beliefs might still be waiting to be challenged? The academic landscape may be in for a transformation. Keep your eyes peeled—there's more to come in this evolving narrative of mathematical discovery!